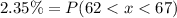Given:
Mean of 52 minutes and a standard deviation of 5 minutes.
Solution:
According to the empirical rule: For the normal distribution, all of the data will fall within three standard deviations of the mean.
1: 68% of the data will fall within the first standard deviation from the mean.
2: 95% of the data fall within two standard deviations.
3: 99.7% of the data fall within three standard deviations.

Therefore, the 7 intervals and their probabilities are:
1.

2.
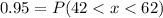
3.
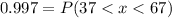
4.
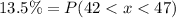
5.
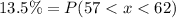
6.

7.