Question

compare two spheres. first has a diameter of 8 yards. The second sphere has a diameter of 1064 yards. Determine the ratio of the volume of the larger sphere to the volume of the smaller sphere

Answer 1

The ratio of the volume of the larger sphere to the volume of the smaller sphere is

2352637 : 1

Explanation:

Volume of a sphere is

`(4)/(3) \pi {r}^(3)`

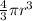
Where r is the radius

radius = diameter / 2

For First sphere

diameter = 8yards

radius = 8 / 2 = 4 yards

Volume of first sphere is

`(4)/(3) \pi( {4})^(3) \\ \\ = (256)/(3) \pi \: {yd}^(3)`

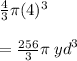
For second sphere

diameter = 1064 yards

radius = 1064 / 2 = 532 yards

Volume of second sphere is

`(4)/(3) \pi( {532})^(3) \\ \\ = (602275072)/(3) \pi \: {yd}^(3)`

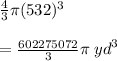
Since the volume of the second sphere is the largest

Ratio of the second sphere to the first one is

`(602275072)/(3) \pi / (256)/(3) \pi \\ \\ = (602275072)/(3) \pi * (3)/(256) \pi \\ \\ = (602275072)/(256) \\ \\ = ( 2352637)/(1) \\ \\ = 2352637: 1`

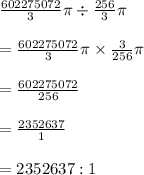
Hope this helps you