Answer:
The given power series
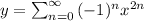 is a solution of the differential equation (1+x^2)y' + 2xy = 0
is a solution of the differential equation (1+x^2)y' + 2xy = 0
Explanation:
This is a very trivial exercise, follow the steps below for the solution:
Step 1: Since n = 0, 1, 2, 3, 4, ........, Substitute the values of n into equation (1) below.
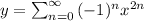 .....................(1)
.....................(1)
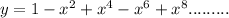
Step 2: Find the derivative of y, i.e. y'
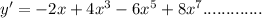
Step 3: Substitute y and y' into equation (2) below:
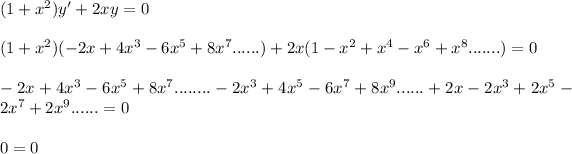
(Verified)
Since the LHS = RHS = 0, the given power series
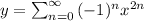 is a solution of the differential equation (1+x^2)y' + 2xy = 0
is a solution of the differential equation (1+x^2)y' + 2xy = 0