Answer:
4
Explanation:
The perimeter of the region is equal to the sum of the perimeter of the four semicircular arc. Since the semicircular arcs have the same measure, therefore:
Perimeter of region = 4 × Perimeter of semicircular arc.
The side of the square = diameter of the semicircle = 2/π.
The radius of semicircle = diameter/2 =
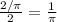
The perimeter of a semicircle = perimeter of a circle ÷ 2
