Question

In a geometric series, the first term is 108 and the common ratio is \frac{2}{3}. Find the sum of the first 8 terms.

Answer 1

311.36

Explanation:

For a geometric series sum of first n terms is
`a(1-r^n)/(1-r)`

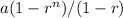
if r is less than 1.

where a is the first term and r is the common ratio.

____________________________\

given

a = 108

r =
`(2)/(3).`

n = 8

thus , sum of n term is

`a(1-r^n)/(1-r)\\=>108(1-(2/3)^8)/(1-2/3)\\=> 108 (1 - 256/6561)/1/3\\=> 108(6561-256)/ 6561/1/3\\=> 108(6305)/2187\\=>311.36`

Thus sum of first 8 terms is 311.36