Answer:
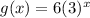
Explanation:
We are given that
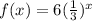
Function f decreases from quadrant 2 to quadrant 1 and approaches y=0
It cut the y- axis at (0,6) and passing through the point (1,2).
Function g(x) approaches y=0 in quadrant 2 and increases into quadrant 1.
It passing through the point (-1,2) and cut the y-axis at point (0,6).
Reflection across y- axis:
Rule of transformation is given by
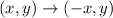
Using the rule then we get

By using
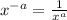
Substitute x=-1

Substitute x=0
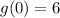
Therefore,
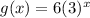 is true.
is true.