Question

Examine the graph showing the half-life of the radioactive isotope substance x.

based on this graph, what might be the best use of this radioactive isotope?

Answer 1

The half-life of radioactive substance X is approximately 5,776 x 10³ years. With a decay constant resembling carbon-14, it's suitable for dating ancient objects, offering reliable age estimations.

1. The decay of the radioactive substance X is described by the equation
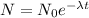`\(N = N_0 e^(-\lambda t)\)`, where N is the number of atoms present,
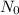`\(N_0\)` is the number of initial atoms,
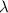`\(\lambda\)` is the decay constant, and t is time.

2. To find the decay constant
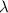`\(\lambda\)`, you use the given data point:
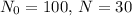`\(N_0 = 100\), \(N = 30\)`, and
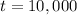`\(t = 10,000\)` years.

3. Substitute these values into the decay equation:
`\(30 = 100 e^(-\lambda \cdot 10,000)\)`.

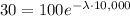4. Solve for
`\(\lambda\): \( \ln(0.3) = -\lambda \cdot 10,000\)`.

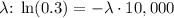5. Calculate
`\(\lambda\): \( \lambda = -(\ln(0.3))/(10,000) \approx 1.2 * 10^(-4)\)`.

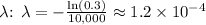6. The average life time
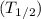`(\(T_(1/2)\))` is given by
`\(T_(1/2) = (\ln(2))/(\lambda)\)`.

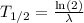7. Substitute the value of
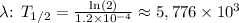`\(\lambda\): \(T_(1/2) = (\ln(2))/(1.2 * 10^(-4)) \approx 5,776 * 10^3\)` years.

This calculated half-life is close to that of carbon-14, indicating its suitability for dating ancient objects.

The half-life of the radioactive isotope substance X is calculated to be approximately 5,776 x 10³ years. This value, similar to the half-life of carbon-14, indicates its potential use for dating ancient objects. The decay of radioactive substances follows an exponential model, and by studying the remaining atoms over time, the half-life provides a crucial parameter for dating applications.

The accuracy of this determination is demonstrated by fitting the decay equation to data points, ensuring reliability in age estimation. The similarity to the half-life of carbon-14, commonly used in archaeology, suggests that substance X could serve as a reliable tool for dating ancient artifacts and materials.

Answer 2

T_{1/2} = 5,776 10³ years

We see this life time as it is very close to the life time of carbon 14, so it could be used for dating ancient objects

Step-by-step explanation:

The radioactive decay of described by an equation of the form

N = N₀
`e^(-\lambda t)`

where N is the number of atoms present, N₀ is the number of initial atoms λ is the activity of the material.

The average life time is defined as the time for which the number of remainng atoms is N = N₀ / 2

`T_(1/2)` = ln 2 /λ

With these expressions, the best method to determine the average life time is to find the activity of the first equation.

For this we look for a point on the graph as accurate as possible,

N₀ = 100, N = 30 and t = 10,000 years

we substitute in the equation

30 = 100 e^{-\lambda 10000}

ln 0.3 = - λ 10000

λ = - (ln 0.3) / 10000

λ = 1.2 10-4

now we can find the average life time

`T_(1/2)` = ln 2 / 1,2 10-4

T_{1/2} = 5,776 10³ years

We see this life time as it is very close to the life time of carbon 14, so it could be used for dating ancient objects