Answer:
At a significance level of 0.05, there is enough evidence to support the claim that there is a significant difference in the proportion of vines infested using Pernod 5 as opposed to Action.
Explanation:
This is a hypothesis test for the difference between proportions.
The claim is that there is a significant difference in the proportion of vines infested using Pernod 5 as opposed to Action.
Then, the null and alternative hypothesis are:
H_0: \pi_1-\pi_2=0\\\\H_a:\pi_1-\pi_2\\eq 0
The significance level is 0.05.
The sample 1 (Pernod 5), of size n1=400 has a proportion of p1=0.06.
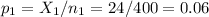
The sample 2, of size n2=400 has a proportion of p2=0.1.
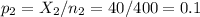
The difference between proportions is (p1-p2)=-0.04.
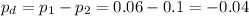
The pooled proportion, needed to calculate the standard error, is:
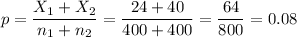
The estimated standard error of the difference between means is computed using the formula:
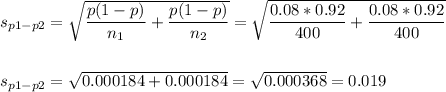
Then, we can calculate the z-statistic as:
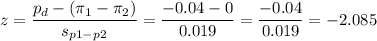
This test is a two-tailed test, so the P-value for this test is calculated as (using a z-table):

As the P-value (0.037) is smaller than the significance level (0.05), the effect is significant.
The null hypothesis is rejected.
There is enough evidence to support the claim that there is a significant difference in the proportion of vines infested using Pernod 5 as opposed to Action.