Question

The springs of a 1700-kg car compress 5.0 mm when its 66-kg driver gets into the driver seat. If the car goes over a bump, what will be the frequency of oscillations?

Answer 1

The frequency of oscillations is 7 Hz

Step-by-step explanation:

Given;

mass of car, = 1700 kg

mass of driver, = 66 kg

compression of the spring, x = 5mm = 0.005 m

The frequency of the oscillation is given as;

`F = (1)/(2 \pi ) \sqrt{(k)/(m) }`

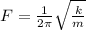
where;

k is force constant

m is the total mass of the car and the driver

m = 1700 kg + 66 kg = 1766 kg

Weight of the car and the driver;

W = mg

W = 1766 x 9.8

W = 17306.8 N

Apply hook's law, to determine the force constant;

F = kx

W = F

Thus, k = W/x

k = 17306.8 / 0.005

k = 3461360 N/m

Now, calculate the frequency

`F = (1)/(2\pi)\sqrt{(k)/(m) } \\\\F = (1)/(2\pi)\sqrt{(3461360)/(1766) }\\\\F = 7 \ Hz`

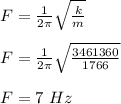
Therefore, the frequency of oscillations is 7 Hz