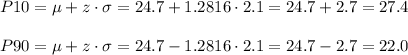Question

Chocolate chip cookies have a distribution that is approximately normal with a mean of 24.7 chocolate chips per cookie and a standard deviation of 2.1 chocolate chips per cookie. Find Upper P 10 and Upper P 90. How might those values be helpful to the producer of the chocolate chip​ cookies?

Answer 1

P10 = 27.4

P90 = 22.0

It helps the producer to know the higher (P10) and lower estimates (P90) for the amount of chocolate chips per cookie.

Explanation:

In P10 and P90 the P stands for "percentile".

In the case of P10, indicates the value X of the random variable for which 10% of the observed values will be above this value X.

In the case of P90, this percentage is 90%.

In this case, we can calculate from the z-values for each of the percentiles in the standard normal distribution.

For P10 we have:

`P(z>z_(P10))=0.1\\\\z_(P10)=1.2816`

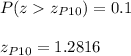
For P90 we have:

`P(z>z_(P90))=0.9\\\\z_(P90)=-1.2816`

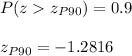
Then, we can convert this values to our normal distribution as:

`P10=\mu+z\cdot\sigma=24.7+1.2816\cdot 2.1=24.7+2.7=27.4 \\\\P90=\mu+z\cdot\sigma=24.7-1.2816\cdot 2.1=24.7-2.7=22.0`