Which of the following pairs of functions are inverses of each other?

Question

asked Aug 11, 2021 27.6k views

2 Answers

Usamec · Answer 1 · 2021-08-14T12:44:11+0000

Answer:

Proved!

Explanation:

For two functions f(x) and g(x) to be inverses of each other then;

f(g(x)) = x and g(f(x)) = x condition must be satisfied.

Checking: A. f(x) = 7x³ + 10 and g(x) = ∛
(x - 10)/(7)

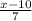
So f(g(x)) = 7(
$\sqrt[3]{(x- 10)/7}$ )³ + 10

We get; 7(
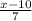(x - 10)/(7) ) + 10 = x - 10 + 10 = x (*This is correct!)

So g(f(x)) =
$\sqrt[3]{((7x^3 + 10) - 10)/7}$

Ten cancels out and we are left with;

$\sqrt[3]{7x^3/7}$ =
$\sqrt[3]{x^3}$ = x (* This is also correct!)