Question

The correlation between the fund returns is 0.1560. What is the expected return and standard deviation for the minimum-variance portfolio of the two risky funds?

Answer 1

The answer is given below

Step-by-step explanation:

The question is not complete. Given that:

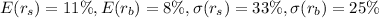`E(r_s)=11\%,E(r_b)=8\%, \sigma(r_s)=33\%,\sigma(r_b)=25\%`, ρ = 0.1560

From the covariance matrix, Cov (B, S) =
`\rho*\sigma_b*\sigma_s=0..1560*33*25=128.7`

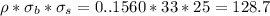
The minimum-variance portfolio is gotten using the formula:

`w_(min)(S)=(\sigma_B^2-Cov(B,S))/(\sigma_S^2+\sigma_B^2-2Cov(B,S))=((25^2)-128.7)/(33^2+25^2-2(128.7))=(625-128.7)/(225+1089-257.4)=0.4697\\\\w_(min)(B)=(\sigma_S^2-Cov(B,S))/(\sigma_S^2+\sigma_B^2-2Cov(B,S))=((33^2)-128.7)/(33^2+25^2-2(128.7))=(1089-128.7)/(225+1089-257.4)=0.9089`

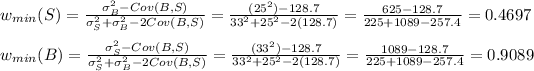
the expected return for the minimum-variance portfolio is:

`E(r_(min))=w_(min)S*E(r_s)+w_(min)B*E(r_b)=11*0.4697+0.9089*8=12.44\%`

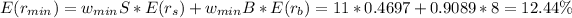
the standard deviation for the minimum-variance portfolio is:

`\sigma_(min)=[w_S^2\sigma_s^2+w_B^2\sigma_B^2+2w_Bw_SCov(B,S)]^(1)/(2) =[0.4687^2*33^2+0.9089^2*25^2+2*0.9089*0.4687*128.7]^(1)/(2)=29.41\%`