Answer:
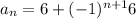
Explanation:
The given sequence is

Assume that the pattern continues as indicated, the we need to find an explicit formula for the above sequence.
From the given sequence it is clear that all odd terms are 12 and all odd terms are 0.
Half of 12 is 6.
We know that,

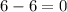
So, the required formula is
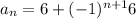
Therefore,
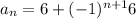 .
.