Answer:
See the explanation below.
Step-by-step explanation:
First find the enthalpies h₁, h₂, h₃, h₄, h₅, and h₆.
Find h₁:
Using Saturated Water Table and given pressure p₁ = 100 kPa
h₁ = 417.5 kJ/kg
Find h₂:
In order to find h₂, add the
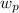 to h₁, where
to h₁, where
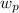 is the work done by pump and h₁ is the enthalpy computed above h₁ = 417.5 kJ/kg.
is the work done by pump and h₁ is the enthalpy computed above h₁ = 417.5 kJ/kg.
But first we need to compute
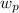 To computer
To computer
Pressures:
p₁ = 100 kPa
p₂ = 15,000 kPa
and
Using saturated water pressure table, the volume of water
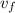 = 1.0432
= 1.0432
Dividing 1.0432/1000 gives us:
Volume of water = v₁ = 0.001043 m³/kg
Compute the value of h₂:
h₂ = h₁ + v₁ (p₂ - p₁)
= 417.5 kJ/kg + 0.001043 m³/kg ( 15,000 kPa - 100 kPa)
= 417.5 + 0.001043 (14900)
= 417.5 + 15.5407
= 433.04 kJ/kg
Find h₃
Using steam table:
At pressure p₃ = 15000 kPa
and Temperature = T₃ = 450°C
Then h₃ = 3159 kJ/kg
The entropy s₃ = 6.14 kJ/ kg K
Find h₄
Since entropy s₃ is equal to s₄ So
s₄ = 6.14 kJ/kgK
To compute h₄
s₄ =
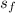 +
+
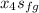

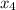 = 6.14 - 2.45 / 3.89
= 6.14 - 2.45 / 3.89
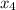 = 0.9497
= 0.9497
The enthalpy h₄:
h₄ =
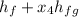
= 908.4 + 0.9497(1889.8)
= 908.4 + 1794.7430
= 2703 kJ/kg
This can simply be computed using the software for steam tables online. Just use the entropy s₃ = 6.14 kJ/ kg K and pressure p₄ = 2000 kPa
Find h₅
Using steam table:
At pressure p₅ = 2000 kPa
and Temperature = T₅ = 450°C
Then h₅ = 3358 kJ/kg
Find h₆:
Since the entropy s₅ = 7.286 kJ/kgK is equal s₆ to So
s₆ = 7.286 kJ/kgK = 7.29 kJ/kgK
To compute h₆
s₆ =
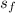 +
+
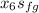
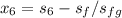
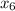 = 7.29 - 1.3028 / 6.0562
= 7.29 - 1.3028 / 6.0562
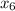 = 0.988
= 0.988
The enthalpy h₆:
h₆ =
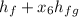
= 417.51 + 0.988 (2257.5)
= 417.51 + 2230.41
h₆ = 2648 kJ/kg
This can simply be computed using the software for steam tables online. Just use the entropy s₅ = 7.286 kJ/kgK and pressure p₅ = 2000 kPa
Compute power used by pump:
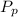 is found by using:
is found by using:
mass flow rate = m = 1.74 kg/s
Volume of water = v₁ = 0.001043 m³/kg
p₁ = 100 kPa
p₂ = 15,000 kPa
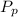 = ( m ) ( v₁ ) ( p₂ - p₁ )
= ( m ) ( v₁ ) ( p₂ - p₁ )
= (1.74 kg/s) (0.001043 m³/kg) (15,000 kPa - 100 kPa)
= (1.74 kg/s) (0.001043 m³/kg) (14900)
= 27.04
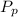 = 27 kW
= 27 kW
Compute heat added
 and heat rejected
and heat rejected
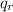 from boiler using computed enthalpies:
from boiler using computed enthalpies:
 = ( h₃ - h₂ ) + ( h₅ - h₄ )
= ( h₃ - h₂ ) + ( h₅ - h₄ )
= ( 3159 kJ/kg - 433.04 kJ/kg ) + ( 3358 kJ/kg - 2703 kJ/kg )
= 2726 + 655
= 3381 kJ/kg
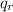 = h₆ - h₁
= h₆ - h₁
= 2648 kJ/kg - 417.5 kJ/kg
= 2232 kJ/kg
Compute net work
W
 =
=
 -
-
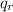
= 3381 kJ/kg - 2232 kJ/kg
= 1150 kJ/kg
Compute power produced by the cycle
mass flow rate = m = 1.74 kg/s
W
 = 1150 kJ/kg
= 1150 kJ/kg
P = m * W

= 1.74 kg/s * 1150 kJ/kg
= 2001 kW
Compute rate of heat transfer in the reheater
Q = m * ( h₅ - h₄ )
= 1.74 kg/s * 655
= 1140 kW
Compute Thermal efficiency of this system
μ
 = 1 -
= 1 -
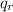 /
/

= 1 - 2232 kJ/kg / 3381 kJ/kg
= 1 - 0.6601
= 0.34
= 34%