A sector of a circle has an arc length of 8 pi inches and a central angle of 20 degrees, as shown. What is the circumference of the circle? Express the answer in terms of Pi.

Question

asked Sep 19, 2021 229k views

2 Answers

Kolchuga · Answer 1 · 2021-09-23T12:01:48+0000

Answer:

Explanation:

Arc length has a formula of

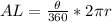$AL=(\theta)/(360)*2\pi r$ and we have all the info we need to solve for the radius, which we will then use in the circumference formula:

$8\pi=(20)/(360)*2\pi r$ and simplifying a bit,

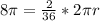$8\pi=(2)/(36)*2\pi r$ and a bit more,

$8\pi=(4\pi r)/(9)$ . Multiply both sides by 9:

72π = πr . Divide both sides by π to get that

r = 72.

Now we will sub that into the circumference formula to find the circumference. Recall that C = 2πr.

C = 2π(72) so

C = 144π