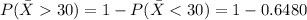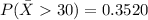Question

The United States Bureau of Labor Statistics (BLS) conducts the Quarterly Census of Employment and Wages (QCEW) and reports a variety of information on each county in America. In the third quarter of 2016, the QCEW reported the total taxable earnings, in millions, of all wage earners in all 3222 counties in America.

Suppose that James is an economist who collects a simple random sample of the total taxable earnings of workers in 56 American counties during the third quarter of 2016. According to the QCEW, the true population mean and standard deviation of taxable earnings, in millions of dollars, by county are ?=28.29 and ?=33.493, respectively.
Let X be the total taxable earnings, in millions, of all wage earners in a county. The mean total taxable earnings of all wage earners in a county across all the counties in James' sample is x??.
Use the central limit theorem (CLT) to determine the probability P that the mean taxable wages in James' sample of 56 counties will be less than $33 million. Report your answer to four decimal places.
P(x??<33)=
Use the CLT again to determine the probability that the mean taxable wages in James' sample of 56 counties will be greater than $30 million. Report your answer to four decimal places.
P(x??>30)=

Answer 1

1

The probability is
`P(\= X < 33) = 0.8531`

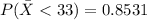
2

The probability is
`P(\= X > 30) = 0.3520`

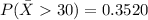
Explanation:

From the question we are told that

The population mean is
`\mu = 28.29`

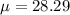
The standard deviation is
`\sigma = 33.493`

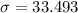
The sample size is
`n = 56`

Generally the standard error for the sample mean
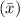`(\= x )` is mathematically evaluated as

`\sigma _(\=x) = (\sigma)/(√(n) )`

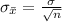
substituting values

`\sigma _(\=x) = (33.493)/(√(56) )`

`\sigma _(\=x) = 4.48`

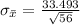
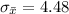
Apply central limit theorem[CLT] we have that

`P(\= X < 33) = [z < (33 - \mu )/(\sigma_(\= x)) ]`

substituting values

`P(\= X < 33) = [z < (33 - 28.29 )/(4.48) ]`

`P(\= X < 33) = [z < 1.05 ]`

From the z-table we have that

`P(\= X < 33) = 0.8531`

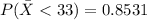
For the second question

Apply central limit theorem[CLT] we have that

`P(\= X > 30 ) = [z > (30 - \mu )/(\sigma_(\= x)) ]`

substituting values

`P(\= X < 33) = [z > (30 - 28.29 )/(4.48) ]`

From the z-table we have that

`P(\= X < 30) = 0.6480`

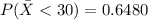
Thus

`P(\= X > 30) = 1- P(\= X < 30) = 1- 0.6480`

`P(\= X > 30) = 0.3520`