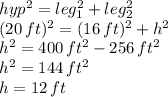Answer:
The pyramid's height h = 12 ft
Explanation:
Notice that the slant height of the pyramid forms a right angle triangle with the segment that joins the bottom end of the slant height with the center of the pyramid's base, and with the pyramid height (h).
The segment joining the slant height with the center of the pyramid's base is one half of the side of the base in length, so that it; 16 feet.
then we have a right angle triangle with hypotenuse given by the pyramid's slant height (20 ft), a leg given by 16 ft, and we need to find the length of the second leg (pyramid's height (h).so we use the Pythagorean theorem: