Question

The dimensions of a cube increase by a scale factor of 2. If the resulting cube has a volume of 64 cm3​, then what was the length of one edge of the original cube?

Answer 1

The original length of the edges are equal to 2 cm.

Explanation:

The volume of a cube is given by the following expression:

`V = a^3`

Where "a" is the length of each edge of the cube. Assuming that the original cube had a length of "x", then its volume would be:

`V_(original) = x^3`

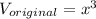
Since the edges of the cube got scaled by a factor of 2, then the new edges are "2*x" and the volume is:

`V_(new) = (2*x)^3\\V_(new) = 8*x^3\\64 = 8*x^3\\x^3 = (64)/(8)\\x^3 = 8\\x = \sqrt[3]{8} = 2`

The original length of the edges are equal to 2 cm.