Question

The waiting times between a subway departure schedule and the arrival of a passenger are uniformly distributed between 0 and 9 minutes. Find the probability that a randomly selected passenger has a waiting time greater than 4.25 minutes.

Answer 1

52.78% probability that a randomly selected passenger has a waiting time greater than 4.25 minutes.

Explanation:

An uniform probability is a case of probability in which each outcome is equally as likely.

For this situation, we have a lower limit of the distribution that we call a and an upper limit that we call b.

The probability that we find a value X greater than x is given by the following formula.

`P(X > x) = (b-x)/(b-a)`

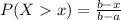
Uniformly distributed between 0 and 9 minutes.

This means that
`a = 0, b = 9`

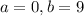
Find the probability that a randomly selected passenger has a waiting time greater than 4.25 minutes.

`P(X > 4.25) = (9 - 4.25)/(9 - 0) = 0.5278`

52.78% probability that a randomly selected passenger has a waiting time greater than 4.25 minutes.