Question

Suppose a random variable x is best described by a uniform probability distribution with range 22 to 55. Find the value of a that makes the following probability statements true.

a. P(X <= a) =0.95
b. P(X < a)= 0.49
c. P(X >= a)= 0.85
d. P(X >a )= 0.89
e. P(1.83 <= x <=a)= 0.31

Answer 1

(a) The value of a is 53.35.

(b) The value of a is 38.17.

(c) The value of a is 26.95.

(d) The value of a is 25.63.

(e) The value of a is 12.06.

Explanation:

The probability density function of X is:

`f_(X)(x)=(1)/(55-22)=(1)/(33)`

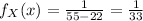
Here, 22 < X < 55.

(a)

Compute the value of a as follows:

`P(X\leq a)=\int\limits^(a)_(22) {(1)/(33)} \, dx \\\\0.95=(1)/(33)\cdot \int\limits^(a)_(22) {1} \, dx \\\\0.95* 33=[x]^(a)_(22)\\\\31.35=a-22\\\\a=31.35+22\\\\a=53.35`

Thus, the value of a is 53.35.

(b)

Compute the value of a as follows:

`P(X< a)=\int\limits^(a)_(22) {(1)/(33)} \, dx \\\\0.95=(1)/(33)\cdot \int\limits^(a)_(22) {1} \, dx \\\\0.49* 33=[x]^(a)_(22)\\\\16.17=a-22\\\\a=16.17+22\\\\a=38.17`

Thus, the value of a is 38.17.

(c)

Compute the value of a as follows:

`P(X\geq a)=\int\limits^(55)_(a) {(1)/(33)} \, dx \\\\0.85=(1)/(33)\cdot \int\limits^(55)_(a) {1} \, dx \\\\0.85* 33=[x]^(55)_(a)\\\\28.05=55-a\\\\a=55-28.05\\\\a=26.95`

Thus, the value of a is 26.95.

(d)

Compute the value of a as follows:

`P(X\geq a)=\int\limits^(55)_(a) {(1)/(33)} \, dx \\\\0.89=(1)/(33)\cdot \int\limits^(55)_(a) {1} \, dx \\\\0.89* 33=[x]^(55)_(a)\\\\29.37=55-a\\\\a=55-29.37\\\\a=25.63`

Thus, the value of a is 25.63.

(e)

Compute the value of a as follows:

`P(1.83\leq X\leq a)=\int\limits^(a)_(1.83) {(1)/(33)} \, dx \\\\0.31=(1)/(33)\cdot \int\limits^(a)_(1.83) {1} \, dx \\\\0.31* 33=[x]^(a)_(1.83)\\\\10.23=a-1.83\\\\a=10.23+1.83\\\\a=12.06`

Thus, the value of a is 12.06.