Answer:
32.6 feet
Explanation:
The computation of the height of the tree is shown below:
Data provided in the question
One position H = 60 degree
Second position L = 20
B = 40 degree
Based on the above information, the calculations are as follows
In triangle ZWX,
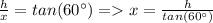
In triangle ZWY

Now from equation 1 and equation 2
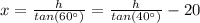
i.e
![20=(h)/(tan(40^(\circ)))-(h)/(tan(60^(\circ))) => h[1.1917535926-0.57735026919]=20](https://img.qammunity.org/2021/formulas/mathematics/college/uchvzrq4a4obbnsvrhaxeo3rfvznzfs9tk.png)
Hence,
![h=(20)/([1.1917535926-0.57735026919])](https://img.qammunity.org/2021/formulas/mathematics/college/lkl9g3iud77rl7ehqw2zd5zz1i91u196zm.png)
= 32.55190728
= 32.6 feet
Hence, the height of the tree is 32.6 feet