Question

Given: ∆ABC is isosceles m∠ACB = 120°, CM = 12 m∠BMC = 60° Find: AB

Answer 1

36

Explanation:

SUIIIIIIIIIIIIIIIIIIIIII

Answer 2

36

Explanation:

As the triangle ABC is isosceles so A and B has the same measures

And, the sum of the angles is 180°.

Therefore 2 times the measure of angle

B plus 120° = 180°,

So, the equation:

2x + 120 = 180

So x = 30

Now we have to apply the law of sin on the triangle BMC as shown below:

`(12)/(\sin30) = (BC)/(\sin 60)`

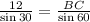
Now calculate for BC

BC = 20.7

We use the law of sin again i.e

`(AB)/(\sin 120) = (20.7)/(\sin 30)`

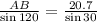
So AB is 36