Answer:
There is a 82% probability that th esample mean annual sales per square foot is at least $384.
Explanation:
We have a population with mean 390 and standard deviation 45.83.
Samples of size n=49 are taken.
The parameters of the sampling distribution are:
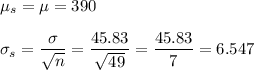
First, we have to calculate the z-score that satisfies:
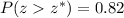
This z-score, looked up in a standard normal distribution table, is z=-0.915.
Then, we can calculate the sample mean as:

There is a 82% probability that th esample mean annual sales per square foot is at least $384.