Answer:
Explanation:
This is very obviously a right triangle problem. Side BO is the hypotenuse of the triangle, measuring 15000'. Side BN is the side adjacent to the given angle B, 55 degrees. This is the cosine ratio:
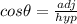
We will fill in what we know:
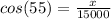 and solving for x:
and solving for x:
15000cos(55) = x
Do that on your calculator in degree mode in exactly that order and get that
x = 8603.6 or, rounded as we need:
x = 8604, choice A.