Answer:
(c)
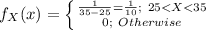
(b) The probability that time taken by Ayesha to prepare breakfast exceeds 33 minutes is 0.20.
(c) The probability that cooking or preparation time is within 2 mins of the mean time is 0.40.
Explanation:
The random variable X follows a Uniform (25, 35).
(a)
The probability density function of an Uniform distribution is:
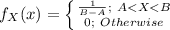
Then the probability density function of the random variable X is:
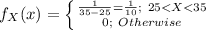
(b)
Compute the value of P (X > 33) as follows:
![P(X>33)=\int\limits^(35)_(33) {(1)/(10)} \, dx \\\\=(1)/(10)\cdot\int\limits^(35)_(33) {1} \, dx \\\\=(1)/(10)* [x]^(35)_(33)\\\\=(35-33)/(10)\\\\=(2)/(10)\\\\=0.20](https://img.qammunity.org/2021/formulas/mathematics/college/nn4rvd826v2rxfzi6hxn696a3k8bnujy9t.png)
Thus, the probability that time taken by Ayesha to prepare breakfast exceeds 33 minutes is 0.20.
(c)
Compute the mean of X as follows:
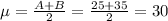
Compute the probability that cooking or preparation time is within 2 mins of the mean time as follows:

![=\int\limits^(32)_(28) {(1)/(10)} \, dx \\\\=(1)/(10)\cdot\int\limits^(32)_(28){1} \, dx \\\\=(1)/(10)* [x]^(32)_(28)\\\\=(32-28)/(10)\\\\=(4)/(10)\\\\=0.40](https://img.qammunity.org/2021/formulas/mathematics/college/fqcf0bccewnid29vbitl0cvym3sb4qatgj.png)
Thus, the probability that cooking or preparation time is within 2 mins of the mean time is 0.40.