Answer:
The margin of error = 2.13
Explanation:
Explanation:-
Given random sample size 'n' =19
mean of the sample(x⁻) = 55 applicants
Given standard deviation of the Population(S.D) = 4
Given confidence intervals are
((52.87.57.14)
we know that The Margin of error is determined by
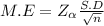
The confidence intervals are determined by
(x⁻ - M.E , x⁻+ M.E)
Step(ii):-
Given confidence intervals are
((52.87.57.14)
Now equating
(x⁻ - M.E , x⁻+ M.E) = ((52.87 , 57.14)
Given mean of the sample x⁻ = 55
( 55 - M.E , 55 + M.E) =((52.87.57.14)
Equating
55 - M.E = 52.87
M.E = 55 - 52.87
M.E = 2.13
Final answer:-
The margin of error = 2.13