Question

Assume the time required to complete a product is normally distributed with a mean 3.2 hours and standard deviation .4 hours. How long should it take to complete a random unit in order to be in the top 10% (right tail) of the time distribution?

Answer 1

Answer: 3.712 hours or more

Explanation:

Let X be the random variable that denotes the time required to complete a product.

X is normally distributed.

`X\sim N(\mu=3.2\text{ hours},\ \sigma=0.4\text{ hours} )`

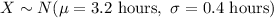
Let x be the times it takes to complete a random unit in order to be in the top 10% (right tail) of the time distribution.

Then,
`P((X-\mu)/(\sigma)>(x-\mu)/(\sigma))=0.10`

`P(z>(x-3.2)/(\sigma))=0.10\ \ \ [z=(x-\mu)/(\sigma)]`

As,
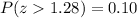`P(z>1.28)=0.10` [By z-table]

Then,

`(x-3.2)/(0.4)=1.28\\\\\Rightarrow\ x=0.4*1.28+3.2\\\\\Rightarrow\ x=3.712`

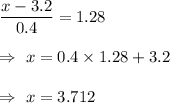
So, it will take 3.712 hours or more to complete a random unit in order to be in the top 10% (right tail) of the time distribution.