Answer:
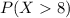
And for this case we can use the cumulative distribution function given by:
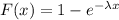
And if we use this formula we got:

Explanation:
For this case we can define the random variable of interest as: "The life of an electric component " and we know the distribution for X given by:
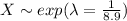
And we want to find the following probability:
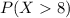
And for this case we can use the cumulative distribution function given by:
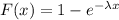
And if we use this formula we got:
