Answer:
(a) The value of E (X) is 4/7.
The value of V (X) is 3/98.
(b) The value of P (X ≤ 0.5) is 0.3438.
Explanation:
The random variable X is defined as the proportion of surface area in a randomly selected quadrant that is covered by a certain plant.
The random variable X follows a standard beta distribution with parameters α = 4 and β = 3.
The probability density function of X is as follows:

Here, B (α, β) is:
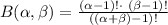
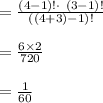
So, the pdf of X is:
![f(x) = (x^(4-1)(1-x)^(3-1))/(1/60)=60\cdot\ [x^(3)(1-x)^(2)];\ 0\leq x\leq 1](https://img.qammunity.org/2021/formulas/mathematics/college/ruarb46hsyuin634f4k909awchl3h9zamx.png)
(a)
Compute the value of E (X) as follows:
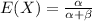

The value of E (X) is 4/7.
Compute the value of V (X) as follows:
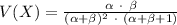
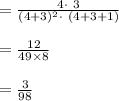
The value of V (X) is 3/98.
(b)
Compute the value of P (X ≤ 0.5) as follows:
![P(X\leq 0.50) = \int\limits^(0.50)_(0){60\cdot\ [x^(3)(1-x)^(2)]} \, dx](https://img.qammunity.org/2021/formulas/mathematics/college/n1mjdpz0kw9f8pq1fuch7f7dicvyt0n26q.png)
![=60\int\limits^(0.50)_(0){[x^(3)(1+x^(2)-2x)]} \, dx \\\\=60\int\limits^(0.50)_(0){[x^(3)+x^(5)-2x^(4)]} \, dx \\\\=60* [(x^4)/(4)+(x^6)/(6)-(2x^5)/(5)]\limits^(0.50)_(0)\\\\=60* [(x^4\left(10x^2-24x+15\right))/(60)]\limits^(0.50)_(0)\\\\=[x^4\left(10x^2-24x+15\right)]\limits^(0.50)_(0)\\\\=0.34375\\\\\approx 0.3438](https://img.qammunity.org/2021/formulas/mathematics/college/qyc9sid3rff00oqb3aqxt6hcqo5zjp3cy0.png)
Thus, the value of P (X ≤ 0.5) is 0.3438.