Question

The shorter leg of a right triangle is 14 feet less than the other leg. Find the length of the two legs of the hypotenuse is 25 feet.

Answer 1

Answer: 9 ft, 23 ft

Explanation:

We know the Pythagorean Theorem is a²+b²=c². Since one leg is 14 less than the other leg, we can use x-14 and the other leg would be x. We can plug these into the Pythagorean Theorem with the given hypotenuse.

(x-14)²+x²=25²

(x²-28x+196)+x²=625

2x²-28x+196=625

2x²-28x-429=0

When we solve for x, we get
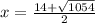`x=(14+√(1054) )/(2)` and
`x=(14-√(1054) )/(2)`.

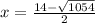Note, since we rounded to 23, the hypotenuse isn't exactly 25, but it gets very close.

Answer 2

9.233 ft, 23.233 ft

Explanation:

If the shorter leg is x, then the longer leg is x+14 and the Pythagorean theorem tells you ...

x^2 + (x +14)^2 = 25^2

2x^2 +28x +196 = 625

x^2 +14x = 214.5

x^2 +14x +49 = 263.5

(x +7)^2 = 263.5

x = -7 +√263.5 ≈ 9.23268

The two leg lengths are √263.5 ± 7 feet, {9.23 ft, 23.23 ft}.