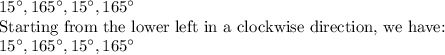Answer:

Explanation:
The ratio of the same side interior angles of two parallel lines is 33:3
When two parallel lines are cut by a transversal, the sum of the same side interior angles is always 180 degrees.
Therefore, the angles are:
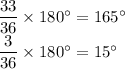
Therefore, the measure of all eight angles formed by the parallel lines and transversal starting from the upper left (can also be seen in the attached diagram) in a clockwise rotation is: