Answer:
1. 58 seconds
2. 5834.5 feet
Explanation:
The equation
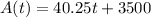 can be used to represent the height of plane A at time t seconds. Similarly, the equation
can be used to represent the height of plane A at time t seconds. Similarly, the equation
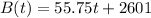 represents the height of plane B after t seconds. To solve for when the planes are at the same height, we can set these two equations equal to get
represents the height of plane B after t seconds. To solve for when the planes are at the same height, we can set these two equations equal to get
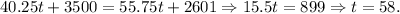 So,
So,
 seconds will pass before the planes are at the same altitude. Plugging in t=58 into either of the equations, we see their altitudes will be
seconds will pass before the planes are at the same altitude. Plugging in t=58 into either of the equations, we see their altitudes will be
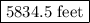 .
.