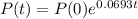Question

The population of a community is known to increase at a rate proportional to the number of people present at time t. If the population has doubled in 10 years, determine the equation that will estimate the population of the community in t years. Let P(0)

Answer 1

`P(t) = P(0)e^(0.0693t)`

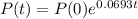
Explanation:

The population of a community is known to increase at a rate proportional to the number of people present at time t.

This means that the population growth is modeled by the following differential equation:

`(dP(t))/(dt) = rP(t)`

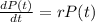
Which has the following solution:

`P(t) = P(0)e^(rt)`

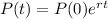
In which P(t) is the population after t years, P(0) is the initial population and r is the growth rate.

The population has doubled in 10 years

This means that
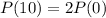`P(10) = 2P(0)`. We use this to find r.

`P(t) = P(0)e^(rt)`

`2P(0) = P(0)e^(10r)`

`e^(10r) = 2`

`\ln{e^(10r)} = ln(2)`

`10r = ln(2)`

`r = (ln(2))/(10)`

`r = 0.0693`

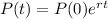
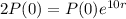
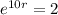
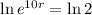
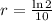
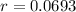
So the equation that will estimate the population of the community in t years is:

`P(t) = P(0)e^(0.0693t)`