Question

A manufacturer sells 50 boats per month at $25000 per boat, and each month demand is increasing at a rate of 4 boats per month. What is the fastest you could drop your price before your monthly revenue starts to drop

Answer 1

For the revenue per month to drop, the price per boat per month has to drop more than $2,000

Step-by-step explanation:

Given:

Number of boats sold per month = 50

Cost of each boat = $25,000

Each month demand increases at a rate of 4 boats per month.

Required:

Find the fastest price could drop before monthly revenue starts to drop.

Revenue, R = Price × Quantity

R = P × Q

Differntiate both sides with respect to time, t:

`(dR)/(dt) = (dP)/(dt) Q + (dQ)/(dt) P`

`= (dP)/(dt) 50 + 4 * 25,000`

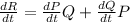
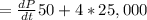
For the fastest price could drop before monthly revenue starts to drop,
`(dR)/(dt) < 0`

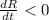
Thus,

`= (dP)/(dt) 50 + 4 * 25,000 < 0`

`= (dP)/(dt) 50 + 100,000 < 0`

`= (dP)/(dt) 50 < -100,000`

`(dP)/(dt) = (-100,000)/(50)`

`(dP)/(dt) = -2,000`

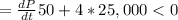
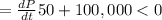
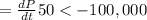
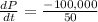
Since the answer is negative, it indicates a drop in price.

Therefore, for the revenue per month to drop, the price per boat per month has to drop more than $2,000