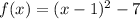Question

What is this function written in vertex form?

The image shows a geometric representation of the function
f(x) = x2 - 2x - 6 written in standard form.
Of(x) = (x –1)2 – 7
O f(x) = (x +1)2-7
O f(x) = (x –1)2-5
O f(x) = (x +1)2 – 5

Answer 1

Answer: A

Explanation:

According to the response above

Answer 2

`f(x) = (x-1)^(2) -7` is the correct answer.

Explanation:

Given that function f(x) is:

`f(x) = x^(2) -2x-6`

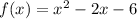
f(x) is a quadratic function in x, meaning that it has a maximum power of 2 of x.

Vertex form of quadratic function is given as:

`f (x) = a(x - h)^2 + k`

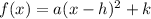
i.e. we make whole square of terms of
`x`.

Now, let us try to make whole square term of
`x`.

`f(x) = x^(2) -2x-6`

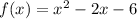
Adding and subtracting 1 from RHS:

`f(x) = x^(2) -2x-6+1-1\\f(x) = (x^(2) -2x+1)-1-6\\f(x) = (x^(2) -2* x* 1+1^2)-7`

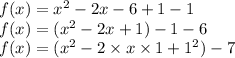
Now, using the formula:

`(a-b)^2 = a^2 -2ab+b^2`

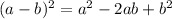
The given function becomes:

`f(x) = (x-1)^(2)-7`

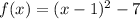
It is comparable to vertex form i.e.
`f (x) = a(x - h)^2 + k`

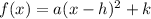
where a = 1, h = 1 and k = -7

Hence, the vertex form of given function is:

`f(x) = (x-1)^(2)-7`