Answer:
a) h(t) = -16t^2 +41t +37
b) see attached (3.270 seconds)
c) (41+√4049)/32 seconds
d) 1.28125 seconds; 63.265625 feet
e) [1.5, 2]: -15; [2, 2.5]: -31; [2.5, 3]: -47
Explanation:
a) The formula and initial values are given. Putting those values into the formula, we get ...
h(t) = -16t^2 +41t +37
__
b) The graph is attached. It shows the t-intercept to be about 3.270 seconds.
__
c) Using the quadratic formula, we can find the landing time as ...
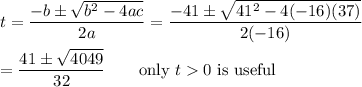
The exact landing time is (41+√4049)/32 seconds.
__
d) The highest point is at t=-b/(2a) = -41/(2(-16)) = 41/32 seconds.
The value of the function at that point is ...
h(41/32) = (-16(41/32) +41)(41/32) +37 = 41^2/64 +37 = 4049/64
The maximum height is 4049/64 = 63.265625 feet.
__
e) For a quadratic function, that average rate of change on an interval is the derivative at the midpoint of the interval. Here, the derivative is ...
h'(t) = -32t +41 . . . in feet per second
Then the average rates of change are ...
arc[1.5, 2] = h'(1.75) = -32·1.75 +41 = -15 ft/s
arc[2, 2.5] = h'(2.25) = -32(2.25) +41 = -31 ft/s
arc[2.5, 3] = h'(2.75) = -32(2.75) +41 = -47 ft/s
These are the average velocity of the water balloon over the given interval(s) in ft/s. Negative indicates downward.