Question

A cube with 1 m on a side is located in the positive x-y-z octant in a Cartesian coordinate system, with one of its points located at the origin. Find the total charge contained in the cube if the charge is given by p_v = x^2 ye^-z mC/m^3

Answer 1

4.61 mC

Step-by-step explanation:

The cube has 1 m side in the positive x-y-z octant in a Cartesian coordinate system, with one of its points located at the origin. The charge density is given as:

`\rho_v=x^2ye^(-z) \ mC/m^3`

Charge density is the charge per unit length or area or volume. It is the amount of charge in a particular region.

The charge Q is given as:

`Q=\int\limits_v {\rho_v} \, dv \\Q=\int\limits_v {\rho_v} \, dv=\int\limits^2_(x=0)\int\limits^2_(y=0)\int\limits^2_(z=0) {x^2ye^(-z)} \, dxdydz\\`

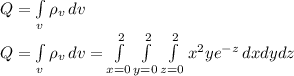
`Q=\int\limits^2_(x=0) {x^2} \, dx \int\limits^2_(y=0) {y} \, dy \int\limits^2_(z=0) {e^(-z)} \, dz \\\\Q=((1)/(3) [x^3]^2_0)((1)/(2) [y^2]^2_0)(-1 [e^(-z)]^2_0)\\\\Q=(-1)/(6) ([x^3]^2_0)( [y^2]^2_0)( [e^(-z)]^2_0)\\\\Q=(-1)/(6)[2^3-0^3][2^2-0^2][e^(-2)-e^0]\\\\Q=(-1)/(6)(8)(4)(0.1353-1)=(-1)/(6)(8)(4)(-0.8647)\\\\Q=4.61\ mC`