Question

The indicated function y1(x) is a solution of the given differential equation. Use reduction of order or formula (5), as instructed, to ?nd a second solution y2(x).

y``+2y`+y=0

Answer 1

Explanation:

We will use the reduction of order to solve this equation. At first, we need a solution of the homogeneus solution.

Consider the equation
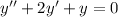`y''+2y'+y=0` We will assume that the solution is of the form
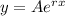`y=Ae^(rx)`. If we plug this in the equation, we get

`Ae^(rx)(r^2+2r+1)=0`

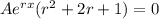
Since the exponential function is a positive function, and A should be different to zero to have non trivial solutions, we get

`r^2+2r+1=0`

By using the quadratic formula, we get the solutions

`r= \frac{-2\pm \sqrt[]{4-4}}{2}=-1`

So one solution of the homogeneus equation is of the form
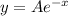`y=Ae^(-x)`. To use the reduction of order assume that

`y = v(x)y_h`

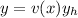
where
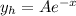`y_h = Ae^(-x)`. We calculate the derivatives and plug it in the equation

`y' = v'y_h+y_h'v`

`y'' = v''y_h+v'y_h'+y_h'v'+y_h''v = v''y_h+2v'y_h+y_h''v`

`(v''y_h+2v'y_h'+y_h''v)+2(v'y_h+y_h'v)+vy_h = 0`

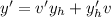
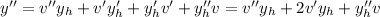
If we rearrange the equation we get

`v''y_h+(2y_h'+2y_h)v'+v(y_h''+2y_h'+y_h)=0`

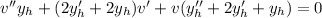
Since
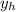`y_h` is a solution of the homogeneus equation we get

`v''y_h+(2y_h'+2y_h)v'=0`

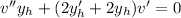
If we take w = v', then w' = v''. So, in this case the equation becomes

`w'y_h+(2y_h'+2y_h)w=0`

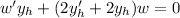
Note that
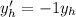`y_h' = -1y_h` so

`w'y_h=0`. Since
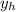`y_h` cannot be zero, this implies

w' =0. Then, w = K (a constant). Then v' = K. So v=Kx+D where D is a constant.

So, we get that the general solution is

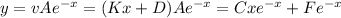`y = vAe^(-x) = (Kx+D)Ae^(-x) = Cxe^(-x) + Fe^(-x)` where C, F are constants.