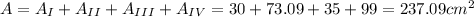Question

Field book of an agricultural land is given in the figure. It is divided into 4 plots . Plot I is a right triangle , plot II is an equilateral triangle, plot III is a rectangle and plot IV is a trapezium, Find the area of each plot and the total area of the field.( use √3 =1.73) plse and mee its urgent

Answer 1

Total area = 237.09
`cm^2`

Explanation:

Area of equilateral triangle is given as:

`A = (\sqrt3)/(4) * side^2`

`\triangle ABC` is equilateral triangle with side = 13 cm

`A_(II) = (\sqrt3)/(4) * 13^2 = 73.09 cm^2`

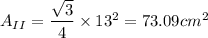
Side EA = ED + DA

CDEF is a rectangle, so CF = ED

EA = CF+DA

19=7+DA

DA = 12 cm

Looking in the region I, i.e. right angle
`\triangle CDA`.

According to pythagoras theorem:

`\text{Hypotenuse}^(2) = \text{Base}^(2) + \text{Perpendicular}^(2)`

`13^(2) = 12^(2) + \text{CD}^(2)\\\text{CD}^(2) = 25\\CD = 5 cm`

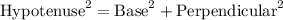
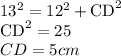
Area of a right angled Triangle:

`A = (1)/(2)* Base * Perpendicular`

`A_I = (1)/(2)* 12 * 5\\A_I = 30 cm^2`

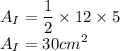
Area of rectangle is given as: Length
`*` Width

`A_(III) = 7 * 5 = 35cm^2`

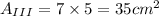
For finding area of trapezium i.e. region IV, let us draw a line parallel to side FG at E that will cut GH at a point P and 'h' is the height of triangle or distance between parallel sides of trapezium.

Now, we have a triangle EPH whose 3 sides are given as a=12, b=9 and c=15.

`s=(a+b+c)/(2)\\\Rightarrow s=(12+9+15)/(2) = 18`

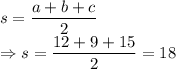
Using hero's formula for area of a triangle:

`A =√(s(s-a)(s-b)(s-c))\\A =√(18(6)(9)(3)) = 54cm^2`

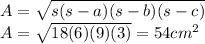
Comparing with:

`A = (1)/(2)* Base * Perpendicular`

`54 = (1)/(2)* 12 * h\\\Rightarrow h = 9cm`

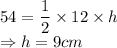
h is distance between parallel sides of trapezium.

Area of trapezium:

`A_(IV) = (1)/(2) (FE+GH)* h\\\Rightarrow A_(IV) = (1)/(2) (5+17)* 9 =99cm^2`

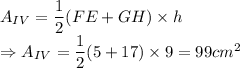
Total area =
`A = A_(I)+A_(II)+A_(III)+A_(IV) = 30 +73.09+35+99 = 237.09cm^2`