Question

A line has a slope of Negative four-fifths. Which ordered pairs could be points on a line that is perpendicular to this line? Select two options.

Answer 1

a. (-2,0) and (2,5)

e. (2,-1) and (10,9)

Explanation:

correct on edge

Answer 2

Following ordered pairs could be on a line perpendicular to the given line:

a. (-2,0) and (2,5)

e. (2,-1) and (10,9)

Question:

Lets complete the question by stating the options of the question first.

Which ordered pairs could be points on a line that is perpendicular to this line?

a. (-2,0) and (2,5)

b. (-4,5) and (4,-5)

c. (-3,4) and (2,0)

d. (1,-1) and (6,-5)

e. (2,-1) and (10,9)

Solution:

Lets find the slope of all the option by using the formula

`m=(y_2-y_1)/(x_2-x_1)`

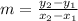
a) m = (5 - 0) / 2 - (-2) = 5/4

b) m = (-5 - 5) / 4 - (-4) = -10/8 = -5/4

c) m = (0 - 4) / 2 - (-3) = -4/5

d) m = (-5 - (-1)) / 6-1 = -4/5

e) m = (9 - (-1)) / 10-2 = 10/8 = 5/4

If 2 lines are perpendicular, the product of their slopes is -1

If a line has a slope of -4/5, we'll multiply it with the slope found for each option. The options in which we get -1 as an answer will be perpendicular to the given line

For option A

Product of Slopes = (-4/5) · (5/4) = -1

Hence the condition holds.

For option B

Product of Slopes = (-4/5) · (-5/4) = 1

Hence the condition does not hold.

For option C

Product of Slopes = (-4/5) · (-4/5) = 16/25

Hence the condition does not hold.

For option D

Product of Slopes = (-4/5) · (-4/5) = 16/25

Hence the condition does not hold

For option E

Product of Slopes = (-4/5) · (5/4) = -1

Hence the condition holds