Question

What is the scale factor in the dilation? On a coordinate plane, triangle A B C has points (4, 4), (7, 8), (10, 4). Triangle A prime B prime C prime has points (10, 10), (17, 20), (25, 10). Two-fifths One-half 2 2 and one-half

Answer 1

2 1/2

Explanation:

Answer 2

2 and one half

Explanation:

Given

ABC = (4,4),(7,8),(10,4)

A'B'C' = (10,10),(17,20),(25,10)

Required:

Find the Scale Factor

Let the scale factor be represented by s

The relationship between ABC, A'B'C' and s is given as

`s(ABC) = A'B'C'`

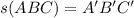
From the given parameters;

A = (4,4) when A' = (10,10)

Using this values;we have

`s * (4,4) = (10,10)`

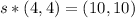
Divide both sides by (4,4)

`(s * (4,4))/((4,4)) = ((10,10))/((4,4))`

`s = ((10,10))/((4,4))`

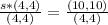
Divide 10 by 4; This gives 2.5

So,

s = 2.5

Using Point B and B'

B = (7,8) when A' = (17,20)

`s * (7,8) = (17,20)`

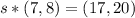
Divide both sides by (7,8)

`(s * (7,8))/((7,8)) = ((17,20))/((7,8))`

`s = ((17,20))/((7,8))`

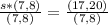
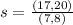
Divide 17 by 7 and 20 by 8; This gives 2.5

So,

s = 2.5

Using Point C and C'

C = (10,4) when C' = (25,10)

`s * (10,4) = (25,10)`

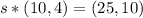
Divide both sides by (10,4)

`(s * (10,4))/((10,4)) = ((25,10))/((10,4))`

`s= ((25,10))/((10,4))`

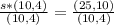
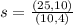
Divide 25 by 10 and 10 by 4; This gives 2.5

So,

s = 2.5

Hence, the scale factor is 2 and one-half(
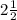`2(1)/(2)`)