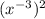Answer:
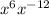 and
and
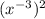
Step-by-step explanation:
Given

Required
Possible Next Step
Open Bracket;
From law of indices;

This implies that
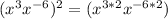
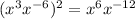
Hence, a possible next step is
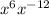
Another possible step is as follows

From law of indices;
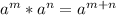
This implies that

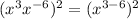
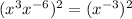
Hence, a possible next step is