Question

A product is introduced to the market. The weekly profit (in dollars) of that product decays exponentially as function of the price that is charged (in dollars) and is given by P ( x ) = 95000 ⋅ e − 0.05 ⋅ x Suppose the price in dollars of that product, x ( t ) , changes over time t (in weeks) as given by x ( t ) = 53 + 0.95 ⋅ t 2 Find the rate that profit changes as a function of time, P ' ( t ) dollars/week How fast is profit changing with respect to time 7 weeks after the introduction. dollars/week

Answer 1

1).
`P'(t) = (-9025t).e^(-0.05(53+0.95t^2))`

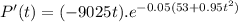
2). (-435.36) dollars per week

Explanation:

Weekly price decay of the product is represented by the function,

P(x) =
`95000.e^(-0.05x)`

And the price of the product changes over the period of 't' weeks is represented by,

x(t) =
`53+0.95t^2`

Function representing the rate of change in the profit with respect to the time will be represented by,

1). P'(t) =
`(dP)/(dx).(dx)/(dt)`

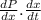
Since, P(x) =
`95000.e^(-0.05x)`

P'(x) =
`95000* (-0.05).e^(-0.05x)`

=
`(-4750).e^(-0.05x)`

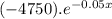
Since, x(t) = 53 + 0.95t²

x'(t) = 1.9t

`(dP)/(dx).(dx)/(dt)=(-4750).e^(-0.05x)* (1.9t)`

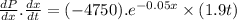
By substituting x = 53 + 0.95t²

`(dP)/(dx).(dx)/(dt)=(-4750).e^(-0.05(53+0.95t^2))* (1.9t)`

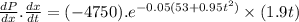
P'(t) =
`(-9025t).e^(-0.05(53+0.95t^2))`

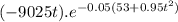
2). For t = 7 weeks,

P'(7) =
`(-9025* 7).e^(-0.05(53+0.95(7)^2))`

=
`(-63175).e^(-4.9775)`

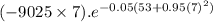
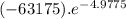
= (-63175)(0.006891)

= (-435.356) dollars per week

≈ (-435.36) dollars per week