Answer:
Correct answer is:
D) ΔHLK ≅ ΔHMN by AAS; ∠K ≅ ∠N because they're corresponding angles in congruent triangles.
Explanation:
We are given the diagram, in which there are 2 triangles namely
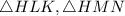 .
.
1. Side KH = Side NH
2.
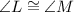
From the given figure, we can derive that:
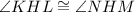
Property used: Vertically opposite angels made by two lines crossing each other are equal.
So, we have two angles (
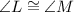 and
and
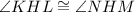 )of the triangle as same and one side equal from the two given triangles
)of the triangle as same and one side equal from the two given triangles
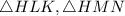 .
.
So, we can say that the two triangles are congruent.
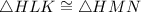
The side is not between the two equal angles, so it is AAS congruence.
And Congruent triangles have their corresponding angles equal.
Therefore, option D) is true :
ΔHLK ≅ ΔHMN by AAS; ∠K ≅ ∠N, because they're corresponding angles in congruent triangles.