Answer:
N = 243.596 N ≈ 243.6 N
Step-by-step explanation:
mass of person = 69 kg ( M )
mass of aluminium ladder = 11 kg ( m )
length of ladder = 6.4 m ( l )
base of ladder = 2 m from the house (d )
center of mass of ladder = 2 m from the bottom of ladder
person on ladder standing = 3 m from bottom of ladder
Calculate the magnitudes of the forces at the top and bottom of the ladder
The net torque on the ladder = o ( since it is at equilibrium )
assuming: the weight of the person( mg) acting at a distance x along the ladder. the weight of the ladder ( mg) acting halfway along the ladder and the reaction N acting on top of the ladder
X = l/2
x = 6.4 / 2 = 3.2
find angle formed by the ladder
cos ∅ = d/l
∅ =
![cos^{-1]](https://img.qammunity.org/2021/formulas/physics/college/t71i5uqp4r0siovbwbykcunbkzj2hp77vc.png) 2/6.4 =
2/6.4 =
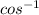 0.3125 ≈ 71.79⁰
0.3125 ≈ 71.79⁰
remember the net torque around is = zero
to calculate the magnitude of forces on the ladder we apply the following formula
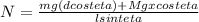
m = 11 kg, M = 69 kg, l = 6.4 , x = 3, teta( ∅ )= 71.79⁰, g = 9.8
back to equation N =
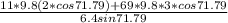
N = (67.375 + 633.938) / 2.879
N = 243.596 N ≈ 243.6 N