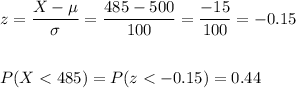Answer:
a. P(X>695)=0.026
b. P(X<485)=0.44
Explanation:
The question is incomplete:
a. higher than 695 on the test.
b. at most 485 on the test.
We have a normal distribution with mean 500 and standard deviation of 100 for the test scores. We will use the z-scores to calculate the probabilties with the standard normal distribution table.
a. We want to calculate the probability that a randomly selected student scores higher than 695.
We calculate the z-score and then we calculate the probability:
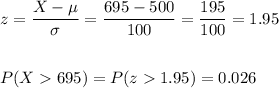
a. We want to calculate the probability that a randomly selected student scores at most 485.
We calculate the z-score and then we calculate the probability: