Question

An aluminium bar 600mm long with a diameter 40mm has a hole drilled in the centre of which 30mm in diameter and 100mm long if the modulus of elasticity is 85GN/M2 calculate the total contraction oon the bar due to comprehensive load of 160KN.

Answer 1

Total contraction on the bar = 1.238 mm

Step-by-step explanation:

Modulus of Elasticity, E = 85 GN/m²

Diameter of the aluminium bar,
`d_(Al) = 40 mm = 0.04 m`

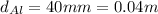
Load, P = 160 kN

Cross sectional area of the aluminium bar without hole:

`A_1 = (\pi d_(Al)^2 )/(4) \\A_1 = (\pi 0.04^2 )/(4)\\A_1 = 0.00126 m^2`

Diameter of hole,
`d_h = 30 mm = 0.03 m`

Cross sectional area of the aluminium bar with hole:

`A_2 = (\pi( d_(Al)^2 - d_(h)^2))/(4) \\A_2 = (\pi (0.04^2 - 0.03^2) )/(4)\\A_2 = 0.00055 m^2`

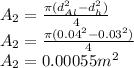
Length of the aluminium bar,
`L_(Al) = 600 mm = 0.6 m`

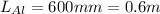
Length of the hole,
`L_h = 100mm = 0.1 m`

Contraction in aluminium bar without hole
`= (P * L_(Al))/(A_1 E)`

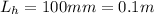
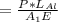
Contraction in aluminium bar without hole
`= (160*10^3 * 0.6)/(0.00126 * 85 * 10^9 )`

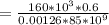
Contraction in aluminium bar without hole = 96000/107100000

Contraction in aluminium bar without hole = 0.000896

Contraction in aluminium bar with hole
`= (P * L_(h))/(A_2 E)`

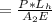
Contraction in aluminium bar without hole
`= (160*10^3 * 0.1)/(0.00055 * 85 * 10^9 )`

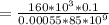
Contraction in aluminium bar without hole = 16000/46750000

Contraction in aluminium bar without hole = 0.000342

Total contraction = 0.000896 + 0.000342

Total contraction = 0.001238 m = 1.238 mm