Question

Pet Place sells pet food and supplies including a popular bailed hay for horses. When the stock of this hay drops to 20 bails, a replenishment order is placed. The store manager is concerned that sales are being lost due to stock outs while waiting for a replenishment order. It has been previously determined that demand during the lead-time is normally distributed with a mean of 15 bails and a standard deviation of 6 bails. The manager would like to know the probability of a stockout during replenishment lead-time. In other words, what is the probability that demand during lead-time will exceed 20 bails

Answer 1

The probability that demand during lead-time will exceed 20 bails is 0.2033.

Explanation:

We are given that it has been previously determined that demand during the lead-time is normally distributed with a mean of 15 bails and a standard deviation of 6 bails.

Let X = demand during the lead-time

So, X ~ Normal(
`\mu=15, \sigma^(2) = 6^(2)`)

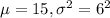The z-score probability distribution for the normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
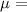`\mu=` population mean demand = 15 bails

`\sigma` = standard deviation = 6 bails

Now, the probability that demand during lead-time will exceed 20 bails is given by = P(X > 20 bails)

P(X > 20 bails) = P(
`(X-\mu)/(\sigma)` >
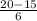`(20-15)/(6)` ) = P(Z > 0.83) = 1 - P(Z
`\leq` 0.83)

= 1 - 0.7967 = 0.2033