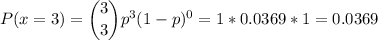Answer:
a. P(x=0)=0.2967
b. P(x=1)=0.4444
c. P(x=2)=0.2219
d. P(x=3)=0.0369
Explanation:
The variable X: "number of meals that exceed $50" can be modeled as a binomial random variable, with n=3 (the total number of meals) and p=0.333 (the probability that the chosen restaurant charges mor thena $50).
The probabilty p can be calculated dividing the amount of restaurants that are expected to charge more than $50 (5 restaurants) by the total amount of restaurants from where we can pick (15 restaurants):
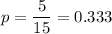
Then, we can model the probability that k meals cost more than $50 as:

a. We have to calculate P(x=0)
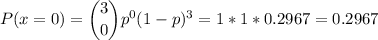
b. We have to calculate P(x=1)
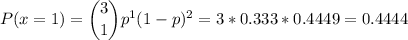
c. We have to calcualte P(x=2)
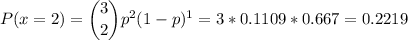
d. We have to calculate P(x=3)