Question

Mark the statements that are true.

A. An angle that measures
radians also measures 30°.
pi/6
O B. An angle that measures pi/3
radians also measures 45°

O C. An angle that measures 180° also measures pi radians.
D. An angle that measures 30° also measures pi/radians.
​

Answer 1

the person abive is correct jzt to confirm

Answer 2

Correct answers:

A. An angle that measures
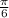`(\pi)/(6)` radians also measures
`30^o`

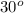
C. An angle that measures
`180^o` also measures
`\pi` radians

Explanation:

Recall the formula to transform radians to degrees and vice-versa:

`\angle\,radians=(\pi)/(180^o) \,* \,\angle degrees\\ \\\angle\,degrees=(180^o)/(\pi) \,* \,\angle radians`

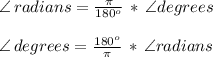
Therefore we can investigate each of the statements, and find that when we have a
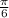`(\pi)/(6)` radians angle, then its degree formula becomes:

`\angle\,degrees=(180^o)/(\pi) \,* \,\angle radians\\\angle\,degrees=(180^o)/(\pi) \,* \,(\pi)/(6) \\\angle\,degrees=(180^o)/(6) \\\angle\,degrees=30^o`

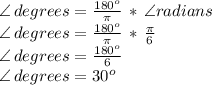
also when an angle measures
`180^o` , its radian measure is:

`\angle\,radians=(\pi)/(180^o) \,* \,\angle degrees\\\angle\,radians=(\pi)/(180^o) \,* \,180^o\\\angle\,radians=\pi`

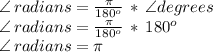
The other relationships are not true as per the conversion formulas